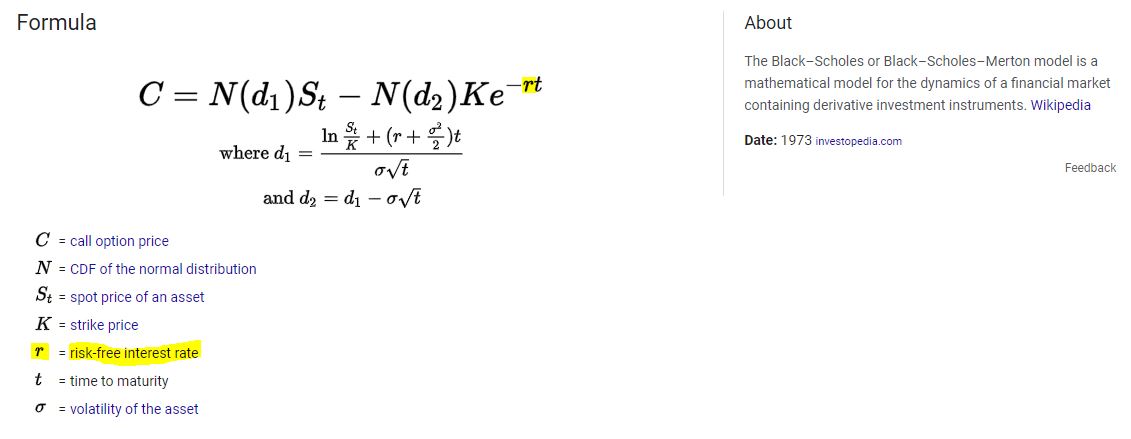Investing in T-bills (Part 9)

The revelation from Part 8 is the hidden cost associated with synthetic long stock (SLS).

To explain this, I now think in terms of an argument that posits edge being arbitraged away once discovered by the masses. T-bills pay annualized interest ~5%: much higher than the default return on cash (currently 0.35% as stated in this second paragraph). This would drive the masses to sell stock, buy calls (or SLS), and invest the capital difference in T-bills. The resultant buying (selling) pressure on calls (puts) would put upward (downward) pressure on call (put) option prices. As seen here, calls end up more expensive than puts.

Nasdaq.com explains this in terms of stock investors who buy on margin:

     > When interest rates rise, the cost of borrowing money to buy
     > stock on margin increases. For example, if you want to buy
     > $10,000 worth of stock on margin, the cost to use margin
     > is much higher when interest rates are at 5% vs. 2%.
     >
     > Therefore, a trader may look to buy a call option to avoid this
     > increased margin interest. For this reason, the pricing of call
     > options will rise when interest rates are hiked…
     >
     > The options market reacts to interest rates, causing call options
     > to increase in value. Instead of buying $10,000 worth of stock
     > on margin, a trader can purchase a call option to benefit from
     > the same upside with much less capital, say $1,500.
     >
     > The saved $8,500 can be put into a risk-free asset such as a
     > T-bill to generate interest, allowing the trader to benefit from
     > the potential upside of a stock and generate income with a bond.
     >
     > Due to the traders’ ability to generate a risk-free return on this
     > saved capital, the price of call options increases. There is no
     > free lunch in the stock market, so when opportunities like this
     > arise, it will reflect in the price of call options.

Investopedia explains the decreased put premiums as follows:

     > Theoretically, shorting a stock with an aim to benefit from a price
     > decline will bring in cash to the short seller. Buying a put has a
     > similar benefit from price declines, but comes at a cost as the put
     > option premium is to be paid. This case has two different
     > scenarios: cash received by shorting a stock can earn interest
     > for the trader, while cash spent in buying puts is interest
     > payable (assuming the trader is borrowing money to buy puts).
     >
     > With an increase in interest rates, shorting stock becomes more
     > profitable than buying puts, as the former generates income
     > and the latter does the opposite. Thus, put option prices are
     > impacted negatively* by increasing interest rates.

Interest rates are actually part of the Black-Scholes model of option pricing. Again, per Investopedia:

This shows call pricing as a function of two complex terms. The second term is subtracted and interest rates (greek letter rho highlighted in yellow) are in the denominator of this second term. As interest rates increase, the denominator increases thereby reducing the second term leaving—since less ends up being subtracted—a higher call price.

I will continue next time.

* — Lower demand can negatively impact put prices.